I pull a block across a surface at constant speed, then double the speed. Does the pulling force also double?

Problem set question:  When you apply a force of 2 N to the right, you cause a wooden block to slide across a rough surface at a constant speed of 3 m/s. Now you want to push the block so it moves at a constant speed of 6 m/s.  How much force should you apply?

(A)          6 N

(B)          4 N

(C)          2 N

(D)          1 N

The most common answer by far, even from a pretty darned skilled class, is (B).  The usual reasoning is tautological:  "If 2 N gives 3 m/s of speed, then I must double the force to double the speed."  Boux.

Of course, I explain in class that Newton never said "F = mv."  He said "F = ma."  Since the acceleration is zero in either case, the pulling force will still equal the friction force.  And since the force of friction doesn't depend on speed, the pulling force will be unchanged.  

Few students believe me.  Only an experiment will convince the class that I'm not full of crap.

So I had Brendan use the experimental setup shown at the top of the post to verify this answer.  A force probe on a PASCO variable speed cart is attached to a string pulling a block along a surface.  First, Brendan set cart to a slow speed, and recorded the force probe reading.  Then, he set the cart to a very fast speed, and recorded the force probe reading.  Results:

This cart was moving slow.

At a slow speed, the force probe reads 0.69 N plus or minus about 0.05 N.

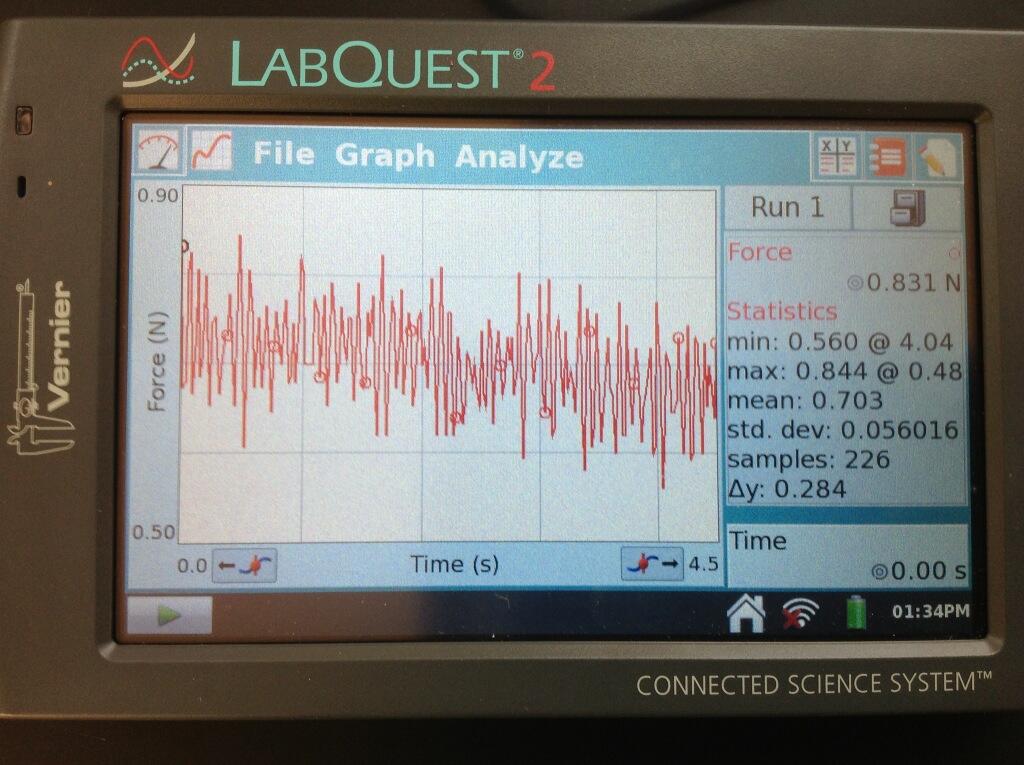This cart was moving fast.
Same pulling force, though...

And at a fast speed, the force probe reads 0.70 N plus or minus about 0.05 N.

Conclusion:  Doubling speed does NOT double force.  I was right: physics works.  Imagine that...