Geometric optics -- meaning, the behavior of light without reference to its wave properties -- can be a fun spring topic for upperclassmen. After the rigors of Newton's laws, after the abstraction of electric and magnetic fields, geometric optics seems straightforward, both mathematically and experimentally. And for a freshman physics class, geometric optics provides an easy-to-verify set of behavioral rules that can be made semi-quantitative.
But what specific parts of geometric optics should you cover? And to what depth?
I've heard positive responses to my "Just the Facts" post about circuits. Teachers have said they appreciated the short list in simple language of exactly what circuit topics to teach at each level of physics. So, here's a similar post about geometric optics. Look down the list to the approximate level that you are teaching.
I've written this list from my own notes -- those of you who teach Regents and AP Physics, please post comments or send emails if something here needs correction, addition, or clarification.
Woodberry Conceptual Physics
1. Light as a ray, definition of normal
2. Light reflects at an angle equal to the angle of incidence.
3. The “index of refraction” tells how much slower light moves in a material than in air
4. When light slows down, the ray bends toward the normal; when light speeds up, the ray bends away from the normal.
5. When light would speed up into a new material, but hits at an angle greater than the critical angle, total internal reflection happens. The value of the critical angle depends on how much the light would speed up.
6. For a concave or convex mirror, parallel rays reflect through (or from) the focal point, and rays through (or toward) the focal point reflect parallel to the principal axis.
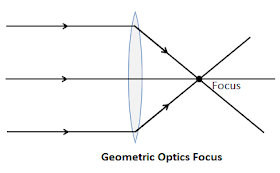
7. For a convex lens, rays through the center are unbent, and parallel rays converge to the far focal point.
8. For a concave lens, rays through the center are unbent, and parallel rays diverge from the near focal point.
9. Categorization of images as upright/inverted, real/virtual, enlarged/reduced
[Those who can figure out on their own how to use Snell’s Law and the thin lens / mirror equation will be eligible for advancement into Honors Physics.]
Regents (please correct me if I screwed up here, New York teachers) / Woodberry Honors Physics:
1. Light as a ray, definition of normal
2. Light reflects at an angle equal to the angle of incidence.
3. The “index of refraction” tells how much slower light moves in a material than in air, n = c/v
4. When light slows down, the ray bends toward the normal; when light speeds up, the ray bends away from the normal, by snell’s law n1sin θ1 = n2sin θ2.
5. When light would speed up into a new material, but hits at an angle greater than the critical angle, total internal reflection happens. The critical angle can be calculated by sin θc = n2/n1.
[Looks like curved mirrors and lenses came off the Regents exam in what, 2002? Honors Physics will include more involved questions than Regents, of course.]
AP Physics B / Woodberry 11th grade general physics / (1990s era Regents):
1. Light as a ray, definition of normal
2. Light reflects at an angle equal to the angle of incidence.
3. The “index of refraction” tells how much slower light moves in a material than in air, n = c/v
4. When light slows down, the ray bends toward the normal; when light speeds up, the ray bends away from the normal, by snell’s law n1sin θ1 = n2sin θ2.
5. When light would speed up into a new material, but hits at an angle greater than the critical angle, total internal reflection happens. The critical angle can be calculated by sin θc = n2/n1.
6a. The focal length of a concave or convex mirror is half the mirror’s radius.
6. For a concave or convex mirror, parallel rays reflect through (or from) the focal point, and rays through (or toward) the focal point reflect parallel to the principal axis.
7. For a convex lens, rays through the center are unbent, and parallel rays converge to the far focal point.
8. For a concave lens, rays through the center are unbent, and parallel rays diverge from the near focal point.
9. Categorization of images as upright/inverted, real/virtual, enlarged/reduced
10. For all converging and diverging lenses, the image distance, object distance, and focal length are related by 1/f = 1/di + 1/do.
11. f is positive for converging lenses and mirrors, negative for diverging.
12. di is positive for real images, negative for virtual
13. In all situations we will deal with, do will be positive
[For #6-13, Regents exams before 2002 provide some wonderful if straightforward sample questions. The AP exam asks more involved questions, and often combines geometric optics with wave optics on the free response.]
looks like you nailed it for the Regents stuff. They took geometric optics out for 2002, and I, and many other NYS teachers, still do curved mirrors and lenses in Regents Physics. I feel that I am doing a disservice to the students if they do not get this in HS and go on to take college physics and it is expected they were exposed to it. The Regents curriculum since 2002 has plenty of time to add in extra topics such as this. I still do some solid state physics, electromagnetic induction, etc. For all the other "old-timers" like me out there, I really value the pre-2002 curriculum and teach many of the topics that were removed. I do not do thermo any more though as it is essentially covered in Chemistry.
ReplyDeleteIn my Honors (not AP) classes, I do everything like Regents with optics, but we do miltiple lenses, or lens-mirror combinations, more with optical instruments, a little more biology applications to the eye...
We are running AP-B next year for the first time (I am not the one teaching it though for specific reasons - my choice). We'll see how we handle geometric optics there...