Big Butt Electricity Fundamentals Quiz

We're just now finishing with electricity concepts -- fields, potentials, resistors, capacitors.  Coincidentally for me, we have a couple days off for "long winter weekend" starting tomorrow.  This is the perfect time to attempt to cement the tough-to-remember rules we've learned over the past month. 

On Monday, I announced the forthcoming "Big Butt Electricity Fundamentals Quiz."  (Why "Big Butt?"  A 17 year old boy is more likely to pay attention to and study for a quiz with this silly, memorable, and quasi-profane name than just another fundamentals quiz.)  The quiz itself is 25 short questions to be done in eight minutes.  Feel free to use it in its entirety, or in portions. 

Introduction to circuits: laboratory exercise

I've already posted about how frustrating electrostatics can be, both to teach and to learn.  Why?  Because I've never found a way to do quantitative, or even qualitative, demonstrations.  Sure, you can hang a balloon from a wall or use an electroscope, but these aren't nearly as satisfying as, say, measuring the pressure at the bottom of a flask to be exactly the 103 kPa you predicted.

I teach circuits immediately after electrostatics.  It's pretty straightforward to do quantitative demonstrations with circuits -- all you need is a voltmeter.  (Or, if you want to be fancy, Vernier voltage and current probes.)  Thing is, I don't do more than a couple quick quantitative demos.  Instead, I run a laboratory exercise over the course of several days.

Especially in the wake of electrostatics, I want the class to get their hands dirty experimentally.  I want them to see, once again, that physics is not a math class, even though the vector addition from electric fields may have given that impression. 

So:  on day one of circuits, I give a crash course of definitions and memorization material.  I write on the board how to deal with series and parallel resistors, including the equivalent resistance formulas and definitions (current through series resistors is the same for each; voltage across parallel resistors is the same for each).  I give one brief example of how to use Ohm's law to deal with a set of two series resistors.

The next day I stop with the theory.  Instead, I show briefly how to use a breadboard to connect resistors to a power supply.  I bring out a large set of labeled resistors, all between 5k and 200k, and a set of voltmeters.  Each student gets a nine-page packet which they are to fill out.  I must sign off on each page before they proceed to the next.  Completing the packet will take about four class periods -- and it's worth every minute.

Here's the first page:

This page takes a while for most folks.  In fact, it might take the entire first class period.  The conversion to microamps should be trivial, but often is not; getting the correct circuit connected on the breadboard is not simple. 

The good news is, the class tends to help each other.  Once one person makes a connection, then that information spreads throughout the class quickly.  I want them to learn from each other as well as from me. 

Once I'm satisfied that a student has both predicted and measured voltage across each resistor, I initial the page and tell the student to move on to the next page.  What's on the next page, you ask?  It's the exact same set of questions... but with a different circuit diagram.  At the end of the post are pictures of the circuits on pages 2-9.

Students work at their own pace.  I ask everyone to get through the first seven circuits over four class periods.  The last two are essentially "extra credit" for the quick workers.  Those who finish early are given time to work on problem set problems, or to help the others in the class. 

Now, I'm pretty picky about the answers to the questions.  If a student says, "The voltage is bigger across the bigger resistor because V=IR," I don't accept that.  "Because V=IR"  is not a reasonable explanation for anything.  For the series resistors, students must indicate that current is the same for each, meaning that bigger R leads to bigger V in Ohm's law.  For the combination circuit, they must show me that they are simplifiying the circuit to a set of series resistors. 

Does this approach work?  Is it worth the four class periods?  I certainly think so.  (If I didn't, I wouldn't do it, right?  :-)  )  I find that my class has performed better on circuit problems on tests and quizzes since I started this exercise about five years ago.  More importantly, when someone does miss a question, we all have a common experience that I can refer to.  "Remember on the circuit lab?  Did the biggest resistor ALWAYS have the biggest voltage?  Remember when the resistors were in parallel?  Oh, yeah..."

GCJ

7

 (Wow, you scrolled down this far?  Awesome.  I'm impressed.  Thanks!)

Mail Time: 5 Steps 2005 vs. 2010 edition

Linda Hoover writes in with a pertinent question:

My oldest son, Daniel used your "5 Steps to a 5 AP Physics B&C" 2005 lime green edition to study for the AP Physics B exam in 2007. He really enjoyed using your book and he in fact, scored a 5! Thank you!

Glad it worked.  Awesome.

My youngest son, Mike is currently taking AP Physics with the plan to take the AP Physics B exam this May. We still have our 2005 version of your bookthat we could use for the review but I noticed that you have 2 later versions, 2008-9 and 2010-11.

Question 1: Have the typos/errors in the [previous versions] been fixed in the latest 2010-11 version?

Yes, as far as I can tell. I don't know whether most of these were my fault or the typesetter's, but the current edition's editor was extremely thorough and (most importantly) knowledgable about physics. I've found one small mistake already that I mentioned and fixed on this blog, but I don't think anything major. (And, I think folks online have exaggerated the frequency of errors. But, whatever, errors are annoying, to readers and to me.)

Question 2: How different is the 2010-11 version from the earlier 2005 copyright?  Should we just use the book we already have (2005) or buy the updated 2010-11 version?

Well, I've made a good deal of editorial changes to the text to make it more readable; plus, we've reordered things to emphasize what's truly important. After the first edition sold well, McGraw-Hill listened more attentively to me about some issues. The figures have been redrawn professionally, the layout is much clearer...

That said, much of the content is similar, and the exams themselves haven't changed. The 2005 book won't hurt, and will save you the 20 beans. If you buy a new one, however, I think I get 25 cents in royalties! :-)

Good luck... let me know if you have comments about the 2010-11 edition.

GCJ

How thick is a door, anyway?

Here's a work-energy theorem problem that I assigned for homework in general physics:

A 6-gram bullet fired through a door enters at 800 m/s and leaves at 600 m/s. If the door material is known to exert an average resistive force of 5600 N on bullets of this type at usual speeds, find the thickness of the door.

While most students did it right, too many were careless in their arithmetic.  The answer as I calculate it is 0.15 m, that is, 15 cm.  I am not concerned if someone gets 20 cm, or 5 cm, or something that reasonably could be the thickness of a door.  Big whoop, they divided wrong on their calculators.  I'm not teaching a class in calculator use, so these students (assuming they approached the problem correctly) get full or nearly full credit.

However, some folks said the door's thickness was 0.001 m, 0.0002 m, and 20 m.  In these students' minds, all they did was make a calculator mistake.  I disagree.  They made a serious physics error.

Part of teaching physics is developing an instinct and undersatnding for the meaning behind numbers.  0.001 m is a millimeter, 0.0002 m is two-tenths of a millimeter -- I've never seen a door this thick.  Or thin.  20 m is the distance across our entire science hallway, so is not door-sized.

How do I insist that students conceptualize the meaning of their numerical answers?  Firstly, ridiculous answers that can be excluded on reasonability grounds lose enormous amounts of credit.  Where I might take off only 1 of 10 points for a minor mistake in a calculation, I will take 3 or 4 points off for a ridiculous answer.

Secondly, I follow up with quizzes wherever possible.  Consider the quiz that's coming tomorrow:

1. A bullet fired through a door enters at 800 m/s and leaves at 600 m/s. Which of the following is possibly the thickness of the door?

   (A) 0.2 m

   (B) 0.002 m

   (C) 0.0002 m

   (D) 2 m

   (E) 20 m

I don't say that one quiz will stop the flow of 2 cm tall people and cars moving 10,000 m/s.  I merely suggest that the cumulative effect of insisting on reasonable answers every day might have the desired effect.

Work done in carrying an object forward

In introducing work to my general physics class, I use the formulation W = F Δx cos θ.  We spend a full day showing how to draw the relevant force vector and the displacement vector tail-to-tail, then use the angle between these as θ.  I find that if students follow this procedure step by step, they're not likely to go wrong; but when they don't carefully draw the force and displacement vectors, or when they have a misconception about the direction of the force, then they can't calculate the work done by that force.

A day or two into the discussion of work, I want to broach the issue of carrying a box across the floor at constant speed.  The work done by the carrying force in this case is ZERO.  But, students are never happy about that answer, nor do they usually come to that answer on their own.

So we approach the problem in baby steps. 

FIRST:  I pick up a 1 kg weight.  I ask the class to answer in their notebooks:  what is the work done by me in lifting the weight 1 m off the ground at constant speed?

After about a minute, I go around the class with starburst candies in my hand.  I give a candy to each student who has drawn, or even attempted to draw, force and displacement vectors.  Whether or not their answer is right, students don't get candy if they haven't drawn and labeled these vectors. 

Since both the force applied by me and displacement point upward, the angle between those vectors is zero.  Thus, the work done by me is (10 N)(1 m)(cos 0) = 10 J.

SECOND:  I put the weight down on the floor.  I ask the class to answer in their notebooks: what is the work done by me in lowering the 1 kg weight a distance of 1 m down to the floor at constant speed?

This time, I give a piece of candy to each student who has CORRECTLY drawn the force and displacement vectors.  The most common mistake here is to draw the force of me pointing down.  I pick one student who made this mistake, hand him the mass, and ask him to push downward on the weight while lowering it at constant speed.  He sees very quickly that he can't do it -- he must apply an upward force.

Then I go over the correct answer.  Since the force of me is upward, and the displacement is downward, the angle between those vectors is 180 degrees.  Thus, the work done by me is (10 N)(1 m)(cos 180) = -10 J.

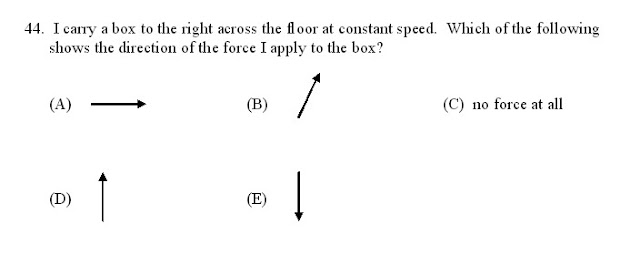
THIRD, usually the next day:  I give the following multiple choice question as part of a quiz:

Of course, the answer is (D).  Since the box moves at constant speed, then the net horizontal force must be zero.  Something doesn't need to experience a rightward force in order to move to the right!  That's an issue we discussed early on in the year... but that most everyone forgot.

Once we can all agree that only an upward force is necessary to move the box to the right, then, finally, I can ask:  how much work is done by me in moving the box to the right at constant speed?  At this point, virtually everyone does it right.  Since the force of me is up and the displacement is right, the angle between these vectors is 90 degrees.  And since cos 90 = 0, I do no work.

Leading questions about the electric field due to point charges

First of all, this blog is up to six -- count 'em, six -- followers.  Woo-hoo!  Only a few million more and I'm as popular as Bill Simmons.  In all seriousness, I'm glad to have everyone aboard.

The diagram at the right is modified a bit from a Serway electrostatics problem.  The goal in the problems I assign, as you may have guessed, is to find the electric field and the electric potential at point P due to the charges.  Today, I just want to talk about finding the electric field.

I will ask this question differently depending on how long we have been studying electrostatics. 

If it is our first foray into fields due to point charges, I will start with:

i.  Is there a charge at point P?

ii.  Is there a force at point P?

iii.  Is there an electric field at point P?

Don't think that's so silly.  I guarantee you, if you don't start here, a large number of students will assume that point P is "positive," or consists of an electron, or will be confused by the sign of point P, or something like that.  Be sure that the class understands the difference between a point charge and a point in space.

If we've had an introduction to fields produced by charges, I might ask the following series of leading questions:

(a) What is the magnitude and direction of the electric field at point P due to the top-left charge?

(b) What is the magnitude and direction of the electric field at point P due to the bottom-right charge?
(c) Calculate the magnitude and direction of the electric field at point P due to BOTH the top-left and bottom-right charges.
(d) What is the magnitude and direction of the electric field at point P due to the bottom-left charge?
(e) Calculate the net electric field at point P. Include both magnitude and direction.

And only after we have gone through example after example in class and on homework would I ask the (nearly) full monty:

1.  Calculate the magnitude and direction of the electric field at point P.

The final, culminating question, which I dread to ask, but I will ask after we are absolutely clear on how to calculate the electric field at point P:

I.  Consider an electron placed at point P.  Calcualte the magnitude and direction of the force experienced by this electron due to the surrounding charges.

Assignment: Write your OWN dang test.

Writing a physics (open response) test question is not in any way an easy task.  It's hard enough to think of a new situation that provides interesting physics, and figure out solvable questions regarding that situation.  It's even harder to write the question so that it's solvable in 10-15 minutes and clear, with no possibility of misinterpretation.

Students get so caught up in the solving of these problems that they fail to appreciate the elegance of their design.  All they see are tough problems to which they have to get the right answers.  It doesn't necessarily occur that, while the details and values are different every time, each problem fits a narrow range of overall themes.  Johnny might be fully capable of dealing with an inelastic collision problem; he might be downright good at projectile problems.  Yet, put him under the pressure of a test, give him an inelastic collision that occurs on the end of a cliff, and ask him how far from the cliff the objects land?  Johnny is likely to be overwhelmed, and freeze like a Microsoft OS with more than one Window open.

One of the many ways I try to debug Johnny is the activity I'm finishing up this week in my general physics course:  the Student-Written Test.  Each student spends lots of time in and out of class writing 4-5 carefully prepared problems for possible use on a test.  I collect these problems, grade them for correctness of solution and quality of presentation, and give them all back.  A day or two later, I give the class a test consisting of three authenticly student-written problems.  (Sure, I adjust wording for clarity, but I use these problems as much verbatim as is reasonable.)

By the end of this one-two week process, my class has developed a serious appreciation for what it takes to write a physics problem... but more importantly, by writing problems of their own, they've become more comfortable dealing with extended multi-topic problems.

Below is the assignment as I handed it out last year, complete with a detailed schedule.  Feel free to use this... I find that I don't usually have time for this sort of thing in AP physics, but the general class can afford to slow the pace.  Besides, what better way to review the topics from the first four months of school?

Problem-writing assignment – instructions and schedule

Your assignment is to write 5 test-quality physics problems, using the concepts we’ve studied so far this year:

Kinematics

Motion graphs

Kinematics in 1-d

Kinematics in 2-d (includes projectile motion)

Newton’s second law

1-D

Newton’s second law in 2-d (includes inclined planes)

Momentum

Impulse-momentum theorem

Law of conservation of momentum

Energy

Definition of work

Work-energy theorem

You don’t have to use all the concepts, but those are all available to you.

A test-quality physics problem is one that involves more than a simple plug-in to an equation. Often, more than one of the above concepts will be required to solve the problem. Looking over past test problems should give you a good idea of the level of complexity you are going for.Writing a test-quality problem is not a simple exercise. As you may have noticed, test problems involve more than simply plugging into an equation. Yet, a test problem must be solvable in 10-15 minutes, so you can’t ask anything horrendously complicated. Finding the balance between solvability and complexity, and meanwhile making every problem interesting, is your challenge.

The structure of a problem will usually follow a standard form. First, you’ll draw and describe a physical situation; then, you’ll ask several different questions about that situation in parts (a), (b), etc. To determine whether your problem has the proper scope and level of difficulty, consider how many of the above physics concepts must be used in the solution. Each problem should involve two or three concepts – no more, no less.

Writing a problem will involve several steps:

1. Think of a situation that provides fertile ground for asking physics questions about it. The situation should be able to be depicted in some sort of picture or diagram, which you’ll need to make (you may draw this freehand or with the aid of a computer drawing program). We’ll call this picture and short, written description of the situation your problem sketch.

2. Next, you’ll want to identify what your problem is asking the solver to find. You’ll also want to come up with reasonable values for whatever given information you are providing the solver, and you’ll want to determine how the question(s) will be asked. This is your draft.

3. Finally, you’ll take your draft and put it into finished form. To do this, you’ll have to check over the draft to make sure you have described the situation adequately, provided all the necessary information, and asked the question(s) clearly and unambiguously. Also, to be absolutely sure you have included everything you need to include, you’ll need to solve the problem yourself. This carefully proofread version, including your written-out solution, is your final problem.

Schedule:

The items due here are in addition to the homework problems assigned for this Monday, Wednesday, and Thursday.

Monday, 1/12: 2 sketches due

Wednesday, 1/14: 1 draft, 1 more sketch (3 total turned in)

Thursday, 1/15: 2 more drafts (3 total turned in), 2 more sketches (5 total)

Friday: 2 more drafts (5 total)

Saturday: 5 final problems

Wednesday, January 21: 45-minute test made from student-written problems

After you hand your final problems in on Thursday, I will look at all of your problems. I will grade each problem on a 5-point scale and return them to you on Monday.

On Wednesday, there will be a three-question test composed from questions you have written. The questions we choose could come from either class. We may do some rewording of the questions for the sake of clarity, but the physics content will be exactly what you wrote, with the solutions you devised.

Electostatics Introduction

Dang, but it's tough to teach electostatics.  The subject is so, so abstract.  I have no quantitative demonstrations; even getting qualitive demonstrations like hanging a balloon from a wall, or showing the repulsion of identically charged styrofoam balls, to work poses a challenge to me in my classroom.  Nevertheless, the AP curriculum demands that I make my best effort.  And, any student who continues into college physics will appreciate whatever exposure to electrostatics that I can give him.

The key to my approach is to AVOID COULOMB'S LAW as if it had occult powers.  Most textbooks start with the evil Law stating the force between two point charges.  Problem is, if you begin with Coulomb's Law, no matter how carefully you handle the presentation, many students will try to use Coulomb's Law for everything.  Got a charged particle hanging from a string in a known electric field?  F = kQQ/r².  Got two parallel plates, want to know the electric field between them?  F = kQQ/r².  Grr.

My solution, which has proven effective for me, is to begin with the definition of an electric FIELD, F = qE.  I spend several days doing nothing but straightforward conceptual questions and simple calculations with this equation.  I hammer over and over, making my students say the words:  Positive charges are forced in the direction of an electric field, negative charges are forced opposite an electric field.  Neither an electric field nor an electric force is ever "negative" -- rather, since both are vectors, we state the magnitude and direction.

The following quiz is given a day or two after we begin the study of electric fields.  Note that only the last question could really be considered at the AP level.  Nevertheless, students regularly bomb this type of quiz until I've given about four similar quizzes.

Next, I try to get the class to understand the difference between a point in space and a charge placed at a point in space.  You think I'm kidding?  See if YOUR class knows the difference.  :-)

1. Write the equation for the force of an electric field.

2. An electric field points right. What is the direction of the electric force on a +3μC charge in this field?

3. An electric field points north. What is the direction of the electric force on an electron in this field?

4. A 500 N/C electric field points left. What is the electric force on a -2 C charge in this field?

5. An electric field points to the right. An electron enters this field moving to the left. In one sentence or less describe the motion of the electron immediately after it enters the field.

6. The charge on an electron is 1.6 x 10-19 C; the mass of a proton is 1.7 x 10-27 kg. A proton is placed in an upward electric field of 200 N/C.

(a) What is the direction of the electric force on the proton?

(b) Which is bigger, the electric force or the gravitational force on the proton?

(c) About how many times bigger is the bigger force?

Three masses connected over a pulley

In my 5 Steps to a 5 AP Physics prep book, page 70 is a review of tension problems, also known as many-body problems.  I give eight different situations in which blocks are connected by ropes.  The goal of each problem is to find the tension(s) in the rope(s), and the acceleration of the system.

The approach that I advocate is to draw a separate free body diagram for each block, then write Newton's second law separately for each block.  The acceleration and tension(s) are solved for by adding the Newton's second law equations together.

Ruth Mickle, of Atlanta, noted yesterday that she gets a different answer to problem 6 than is printed in the book.  I agree -- for whatever reason, the answer in my book is wrong.  Below I give a thorough solution. 

The problem shows three blocks connected by strings over a pulley, as shown at the top of the post.  Given that m is 1.0 kg, the question asks for the tensions and acceleration.

Start by drawing three free body diagrams.  Note that the two ropes will have two tensions; I'll label these T1 and T2.

The acceleration will be toward the heavier blocks.  Thus, the mass m will accelerate upward, and the other masses will accelerate downward.  So when we write Newton's second law, for mass m we'll write "up forces - down forces = ma."  For the other masses, we'll write "down forces - up forces = ma."  Always start Newton's second law in the direction of the acceleration.

T2 - mg = ma          2mg + T1 - T2 = 2ma                       4mg - T1 = 4ma

Now, add 'em up.  Note that the T1s and the T2s will cancel in the addition:

-mg + 2mg + 4mg = 7ma

Solving for a, we get a = (5/7)g, or 7.1 m/s².

Now just plug back into the equations above to find that T2 = 17 N, and T1 = 11 N.